What Are the Different Types of Quadrilaterals? What Properties Do They Have?
A Quadrilaterals is a closed shape created by connecting four points, any three of which are non-col linear in geometry. The name ‘quadrilateral’ comes from the Latin word ‘quadrant’, which means four and ‘Laius,’ which means sides.
Definition of a Quadrilateral
A polygon having four sides, four angles, and four vertices is known as a quadrilateral. When naming a quadrilateral, it’s important to remember the vertices’ order. The following quadrilateral, for example, should be called ABED, ABC, ADC, or ADC.
Because they modify the sequence of vertices in which a quadrilateral is created, it can’t be called ACED or DB AC. ABED is a quadrilateral with four sides (AB, BC, CD, DA), as well as two diagonals (AC and DB).
Types of Quadrilaterals
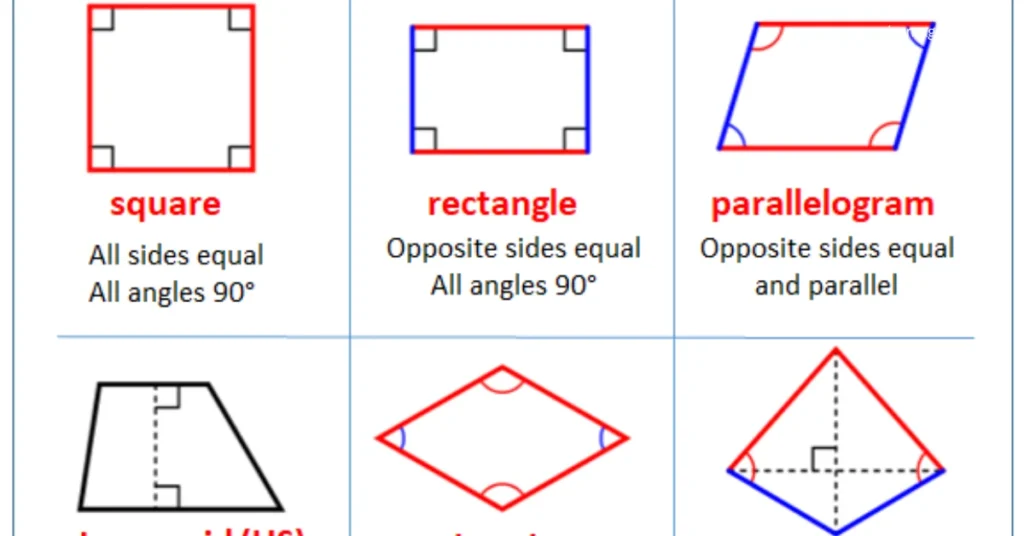
A quadrilateral has four sides, four angles, and four vertices, although the lengths of the sides and angles vary. It’s worth noting that the sum of a quadrilateral’s inner angles is always 360°. The different varieties of quadrilaterals are shown in the table below.
Properties of Quadrilateral
Each of the quadrilaterals mentioned above has its unique set of characteristics. There are, nevertheless, some features that all quadrilaterals share. The following are the details.
- There are four sides to them.
- They are made up of four vertices.
- They are divided into two diagonals.
- 360° is the total of all interior angles.
We’ll go through the additional characteristics of several quadrilaterals in depth. The characteristics of quadrilaterals can be used to identify a quadrilateral.
Square
It’s also known as a rectangle with two neighboring sides that have the same length. A square is a unique circumstance of a rhombus (opposite equal angles, equal sides), It has all of the features of a kite (two sets of adjacent equal sides),
A trapezoid (one pair of opposing sides parallel), a parallelogram (all opposite sides parallel), a quadrilateral or tetrahedron (four-sided polygon), and a rectangle (right-angles, opposite sides equal). They are: A square’s diagonals bisect each other and meet at a 90° angle.
A square’s diagonals cut across its angles. A square’s opposite sides are both parallel and equal in length. The four angles of a square are all the same (360°/4 = 90°, or a straight angle). The four sides of a square are all the same length.
The diagonals of a square are all the same length. The n=2 instance of the n-hypercube and n-porthole families is the square.
Rectangle
Because all of its angles are equal (360°/4 = 90°), it’s also known as an quadrangular quadrilateral or a parallelogram with a right angle. The term oblong is sometimes used to refer to a rectangle that isn’t square. Rectangle is the name for a rectangle having the vertices ABED.
The term rectangle derives from the Latin word rectangular, which combines the words rectums with annulus. If and only if a convex quadrilateral is one of the following, it is a rectangle: at least one right angle in a parallelogram
A parallelogram with equal-length diagonals. A parallelogram ABED with congruent triangles BAD and DC. A quadrilateral with an quadrangular shape. Four right angles form a quadrilateral. A quadrilateral with two equal-length diagonals that intersect each other.
Parallelogram
In Euclidean geometry, a parallelogram is a fundamental quadrilateral having two sets of parallel sides. A parallelogram’s opposing or facing sides are of equal length, and the parallelogram’s opposite angles are of equal measure.
The Euclidean parallel postulate is a direct consequence of the congruence of opposing sides and opposite angles, and neither condition can be demonstrated without using the Euclidean parallel postulate or one of its equivalent formulations.
A trapezoid in American English or a trapezium in British English is a quadrilateral with just one set of parallel sides.
Trapezium
According to Euclidean geometry, a trapezium is a quadrilateral having one pair of parallel opposed sides. Trapezium comes from the Greek word “trapeze,” which meaning “table.” We will learn more about the characteristics of trapezium through examples in this session.
What is the definition of a trapezium? A trapezium is a two-dimensional quadrilateral with a pair of parallel opposing sides (due to its four straight lines). The trapezium’s base and non-parallel sides are known as the trapezium’s base and legs, respectively.
It has four sides and four corners and is a closed planar form. Check if you know this: Which of the following statements are true (T) and which are false (F)?
